8.3 Stoner-Wohlfarth Behavior

First we have done simulations of a particle with a single magnetocrystalline anisotropy axis and a diameter of 60 nm. As expected we find the behavior of a typical Stoner-Wohlfarth particle. The results are shown in Fig. 8.3. Then we have included the demagnetizing field in the simulation and found only a very small influence on the nucleation field, which is reduced by less than 5 %. This is due to the dominating role of the anisotropy, which gives rise to an anisotropy field of more than 13 T. In comparison the demagnetizing field in a particle with perfectly homogeneous magnetization varies from 0.4 to 0.9 T within the particle. This is illustrated in Figs. 8.4, 8.5, and 8.6, where we have plotted the 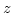$z$-component of the demagnetizing field through different parts of the particle.

Figure 8.3: Nucleation field of an FePt nanoparticle with uniaxial magnetocrystalline anisotropy as a function of the angle of the applied field with respect to the easy axis. The finite element simulation gives the correct result of a Stoner-Wohlfarth particle. If the demagnetizing field is taken into account, the nucleation field is reduced by less than 5 % due to the dominating high magnetocrystalline anisotropy.

Figure 8.4: The 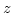$z$-component of the demagnetizing field has been measured along the 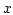$x$-axis through the center of the nanoparticle as shown in the left image. The result is shown in the graph on the right.

Figure 8.5: The 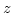$z$-component of the demagnetizing field has been measured along the 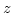$z$-axis through the center of the nanoparticle as shown in the left image. The result is shown in the graph on the right.

Figure 8.6: The 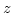$z$-component of the demagnetizing field has been measured along a line parallel to the 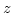$z$-axis close to an edge of the nanoparticle as shown in the left image. The result is shown in the graph on the right.